Geometry 2-5 Guided Practice: Reasoning in Algebra and Geometry
By Matt Richardson
starstarstarstarstarstarstarstarstarstar
Last updated about 3 years ago
11 Questions


10 points
10
10 points
10
Question 2
2.
Assume that a, b, and c are real numbers, that a = b, and that c ≠ 0.
Take Note: Match each item on the left with the property it demonstrates on the right.
Assume that a, b, and c are real numbers, that a = b, and that c ≠ 0.
If 7 = a, then a = 7. | arrow_right_alt | Addition Property of Equality |
a ÷ 4 = b ÷ 4 | arrow_right_alt | Subtraction Property of Equality |
a + 5 = b + 5 | arrow_right_alt | Multiplication Property of Equality |
5(a - b) = 5a - 5b | arrow_right_alt | Division Property of Equality |
-3b = -3a | arrow_right_alt | Reflexive Property of Equality |
If a = b and b = 24, then a = 24. | arrow_right_alt | Symmetric Property of Equality |
a - 17 = b - 17 | arrow_right_alt | Transitive Property of Equality |
b = b | arrow_right_alt | Substitution Property of Equality |
If c = 10, then 10 can replace c in any expression. | arrow_right_alt | Distributive Property |
10 points
10
Question 3
3.
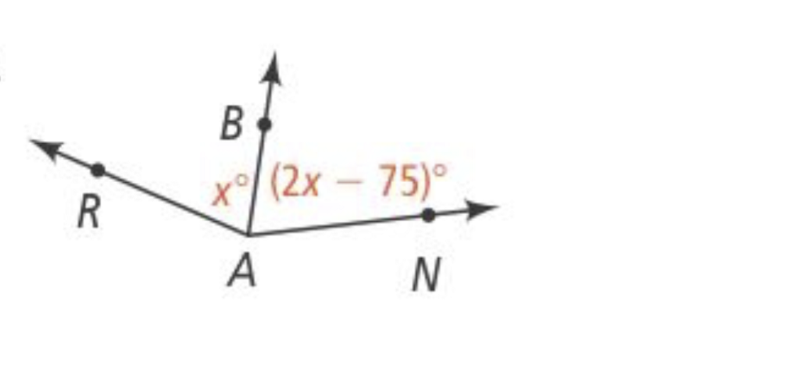
Problem 1 Got It?Given: ray AB bisects ∠RAN
What is the value of x? Justify each step.
Problem 1 Got It?
Given: ray AB bisects ∠RAN
What is the value of x? Justify each step.
10 points
10
Question 4
4.
Take Note: Categorize each item on the left with the property it demonstrates on the right.
- If \overline{PR} \cong \overline{TS} and \overline{TS} \cong \overline{ML}, then \overline{PR} \cong \overline{ML}.
- \angle{1} \cong \angle{1}
- If \angle{B} \cong \angle{M}, then \angle{M} \cong \angle{B}.
- \overline{ST} \cong \overline{ST}
- Reflexive Property of Congruence
- Symmetric Property of Congruence
- Transitive Property of Congruence

10 points
10
Question 5
5.
Problem 2 Got It?
Problem 2 Got It?

10 points
10
Question 6
6.
Problem 2 Got It?
Problem 2 Got It?

10 points
10
Question 7
7.
Problem 2 Got It?
Problem 2 Got It?

10 points
10
Question 8
8.
Problem 2 Got It?
Problem 2 Got It?
10 points
10
Question 9
9.
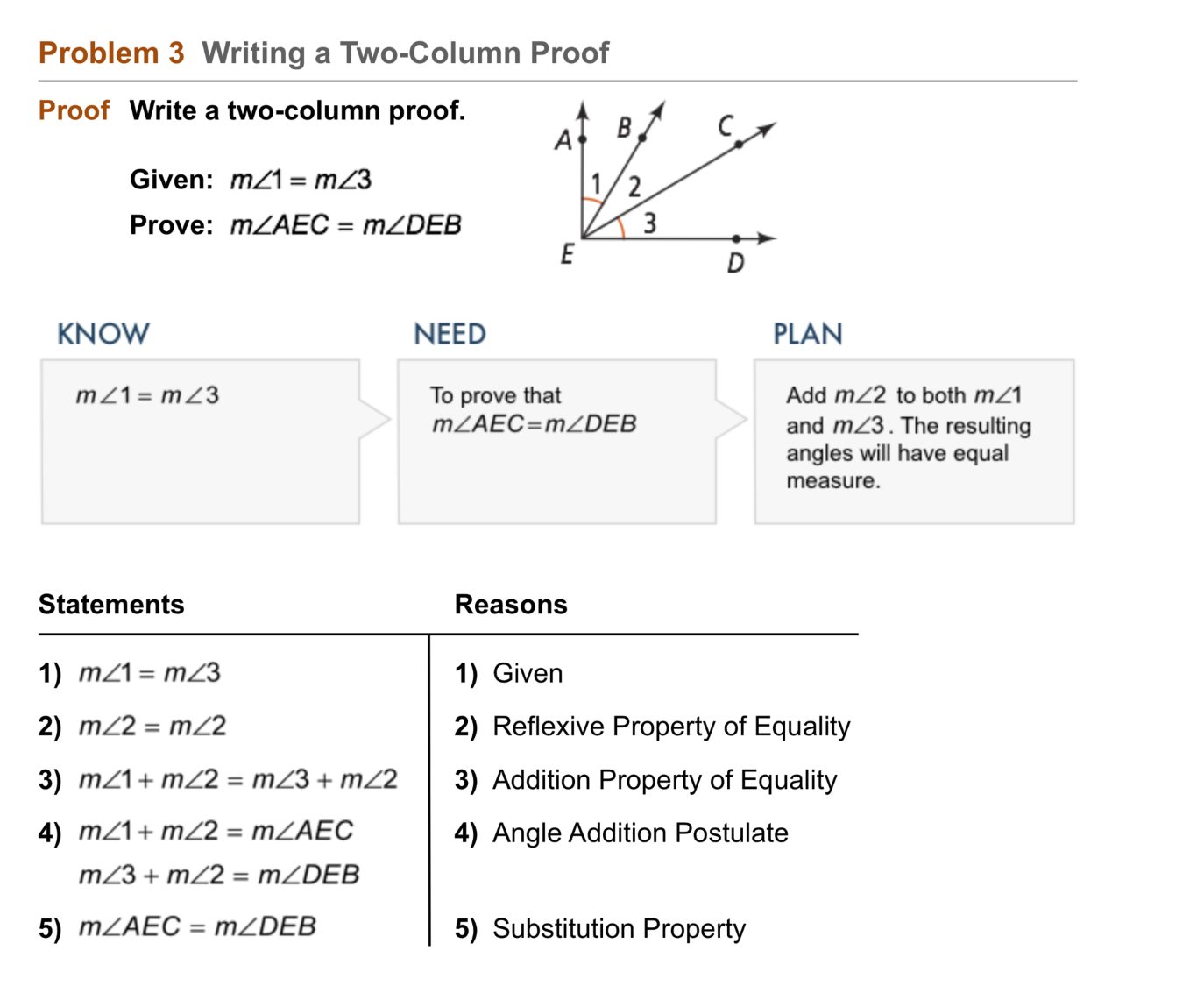
Problem 3 Got It? Complete the two-column proof on the canvas.
You may also complete your work on paper or on a whiteboard and upload a clear picture of it to the canvas.
Problem 3 Got It? Complete the two-column proof on the canvas.
You may also complete your work on paper or on a whiteboard and upload a clear picture of it to the canvas.
10 points
10
Question 10
10.
Problem 3 Got It? Reasoning: In Problem 3, why is Statement 2 necessary in the proof?
Problem 3 Got It? Reasoning: In Problem 3, why is Statement 2 necessary in the proof?
10 points
10
Question 11
11.
Take Note: Summarize the mathematical content of this lesson. What topics, ideas, and vocabulary were introduced?
Take Note: Summarize the mathematical content of this lesson. What topics, ideas, and vocabulary were introduced?