Library

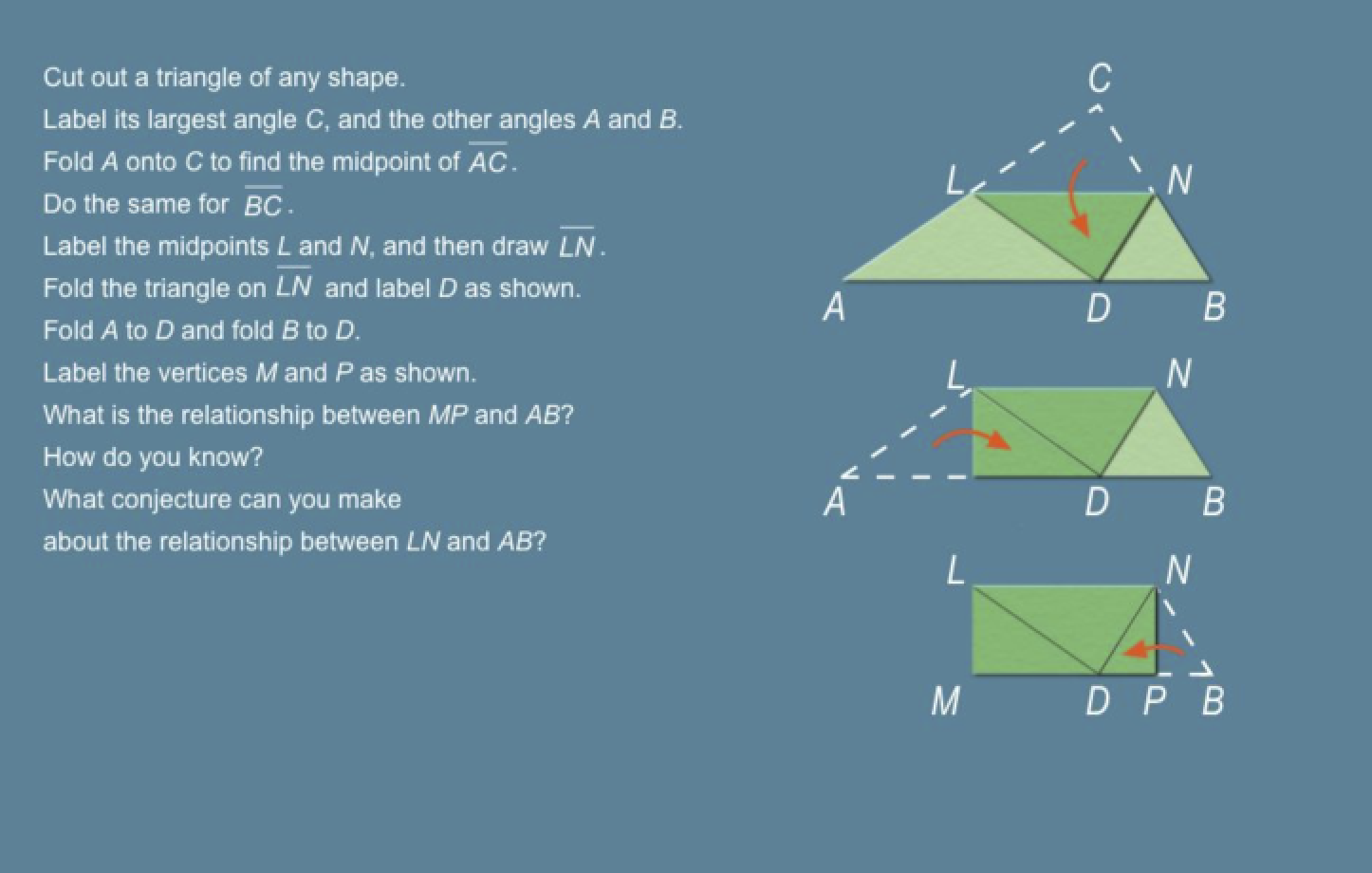
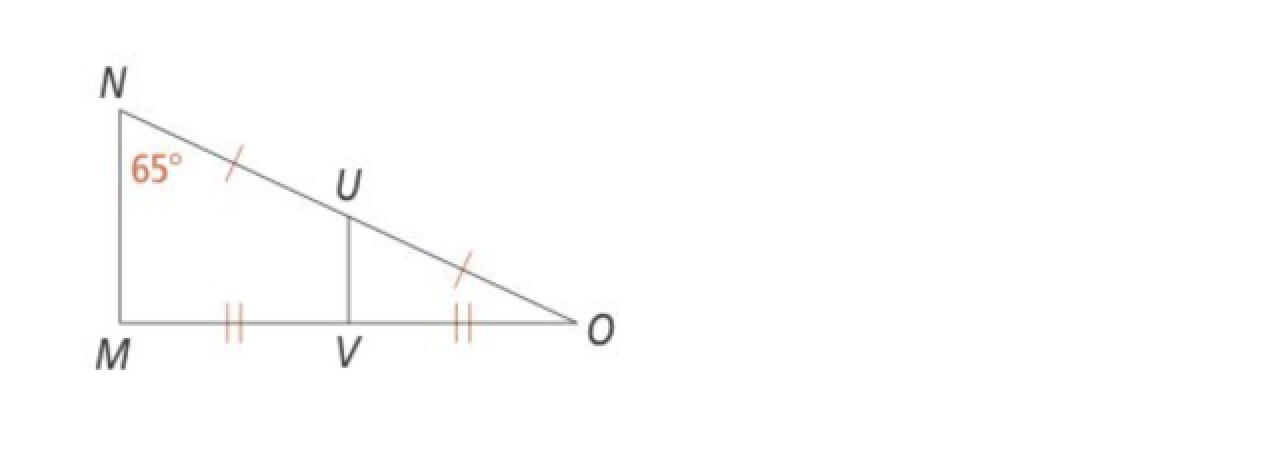
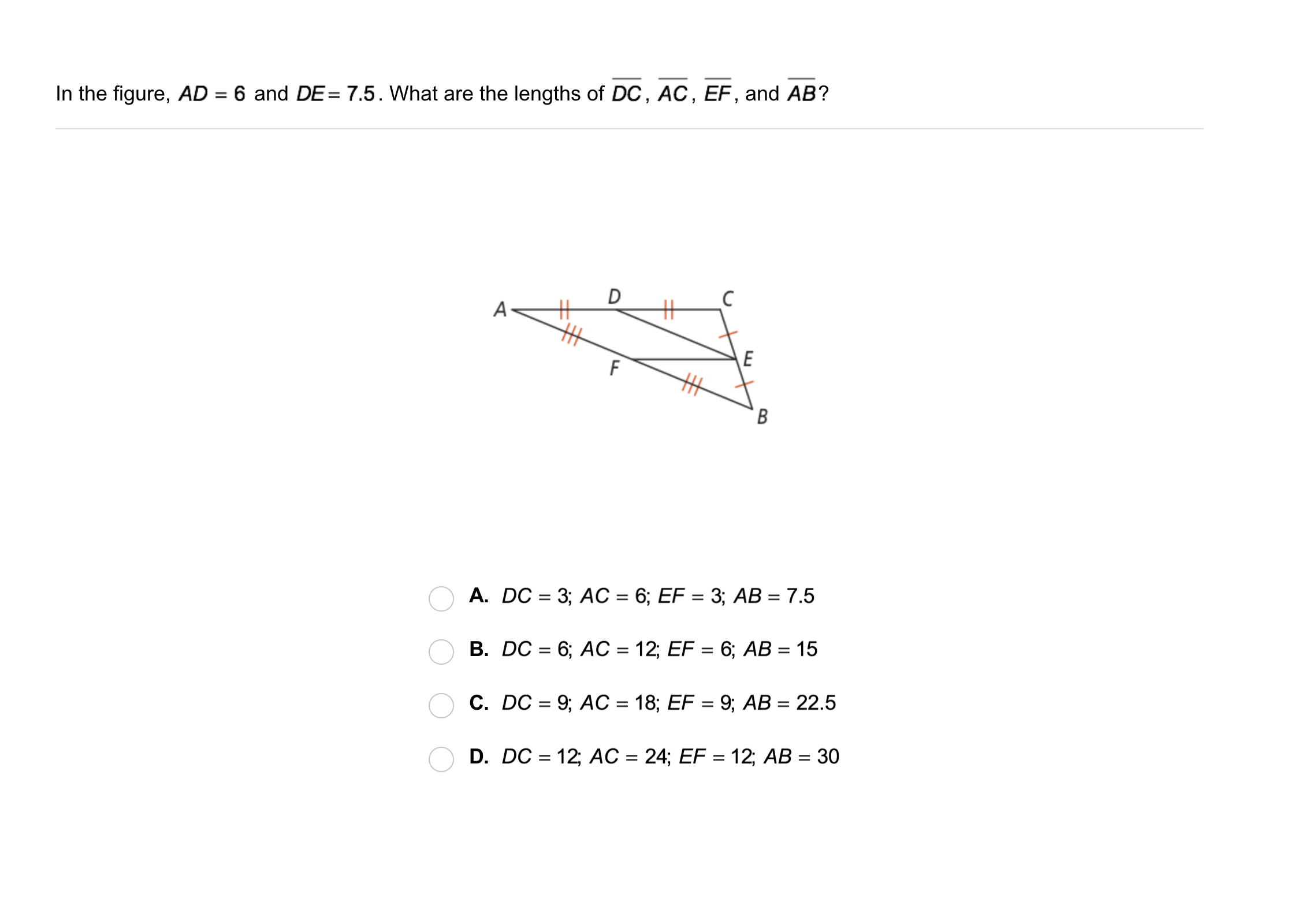
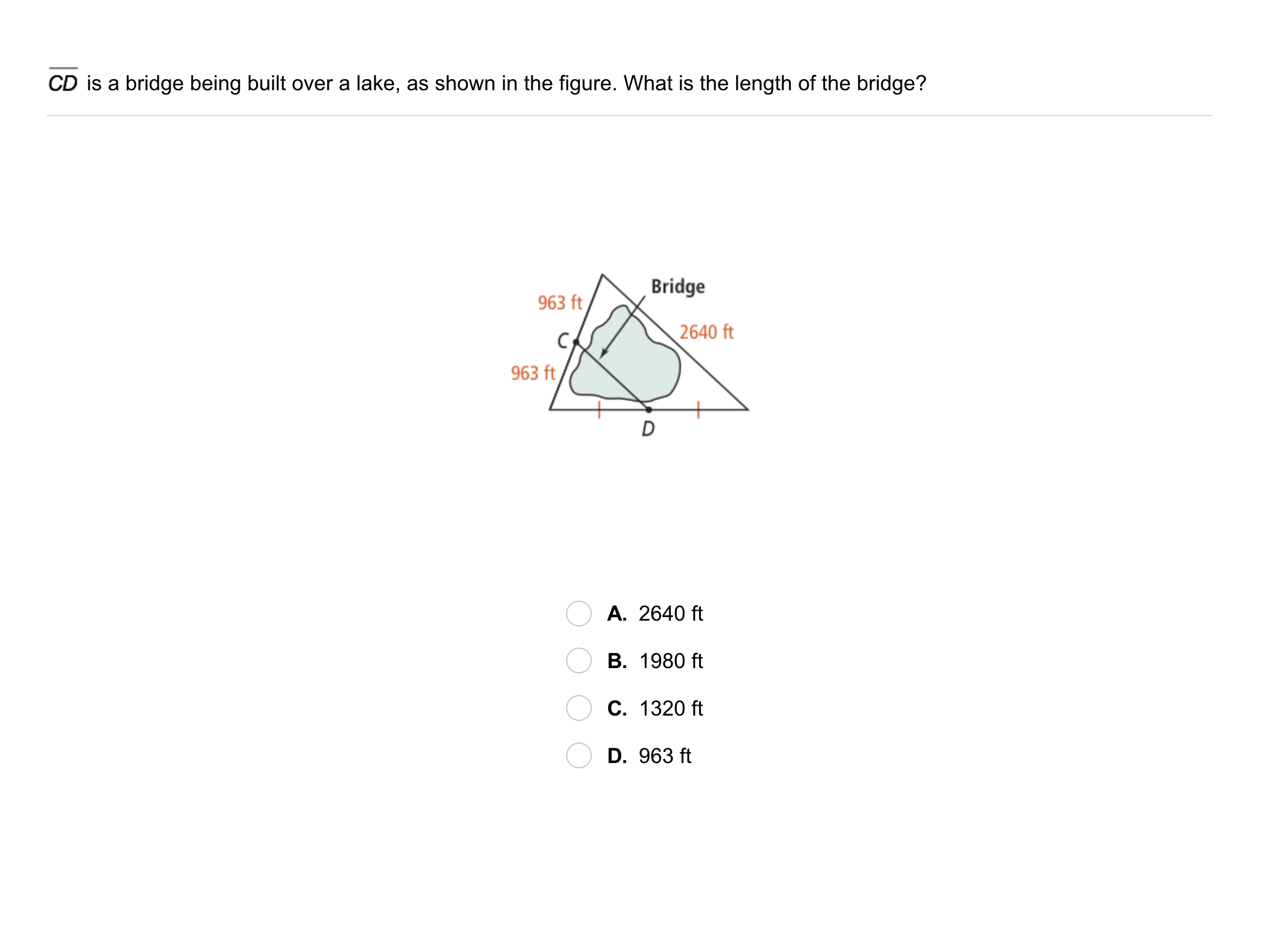
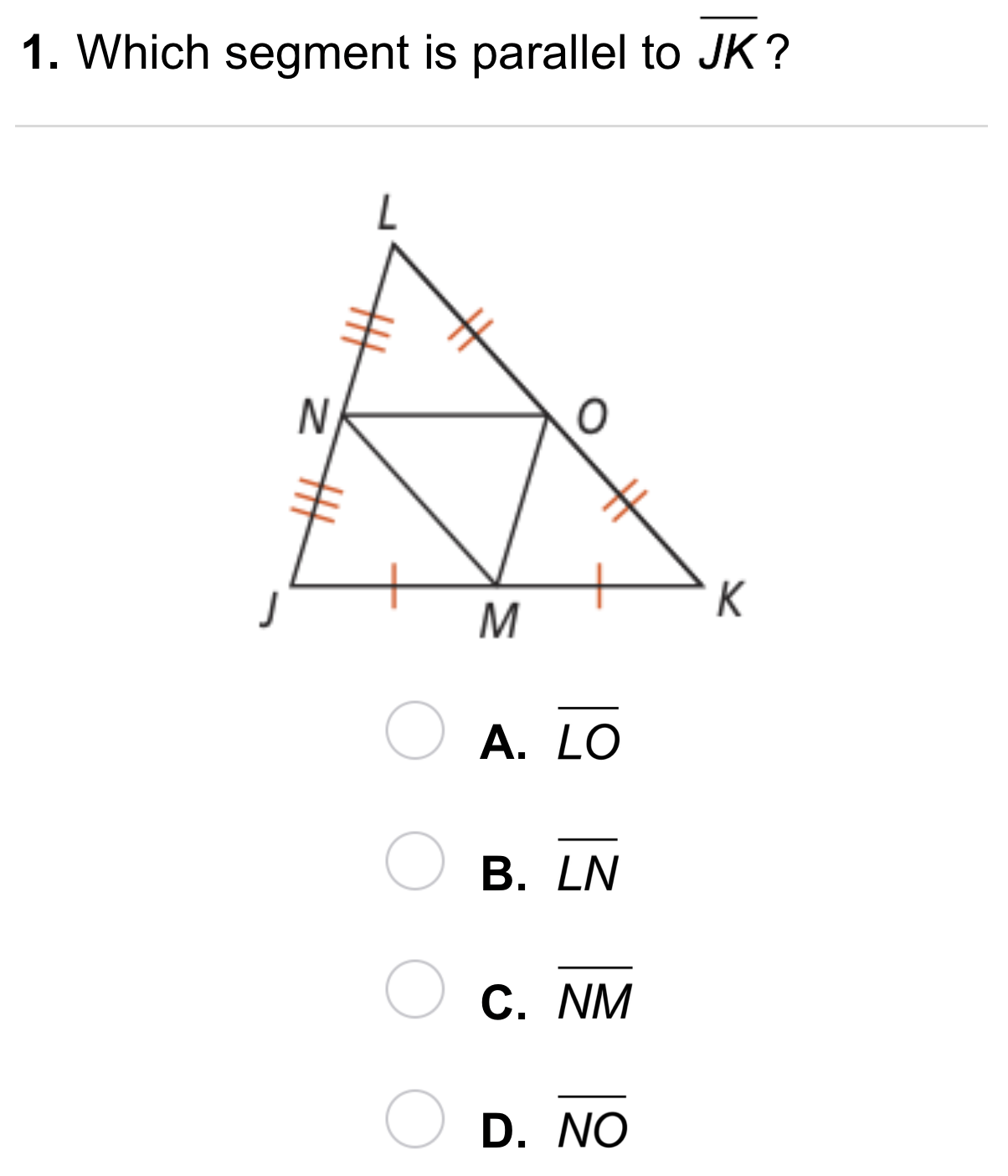
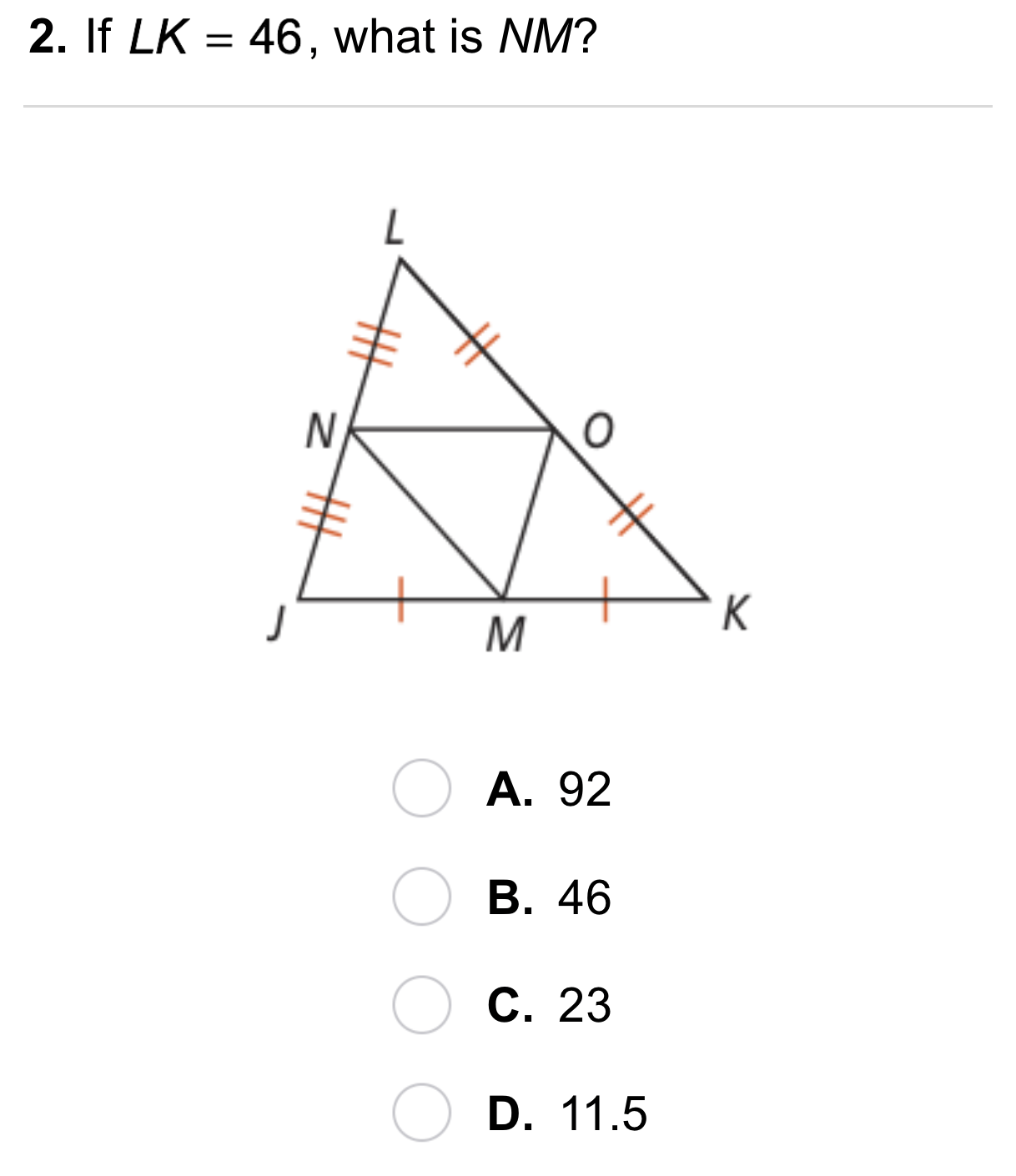
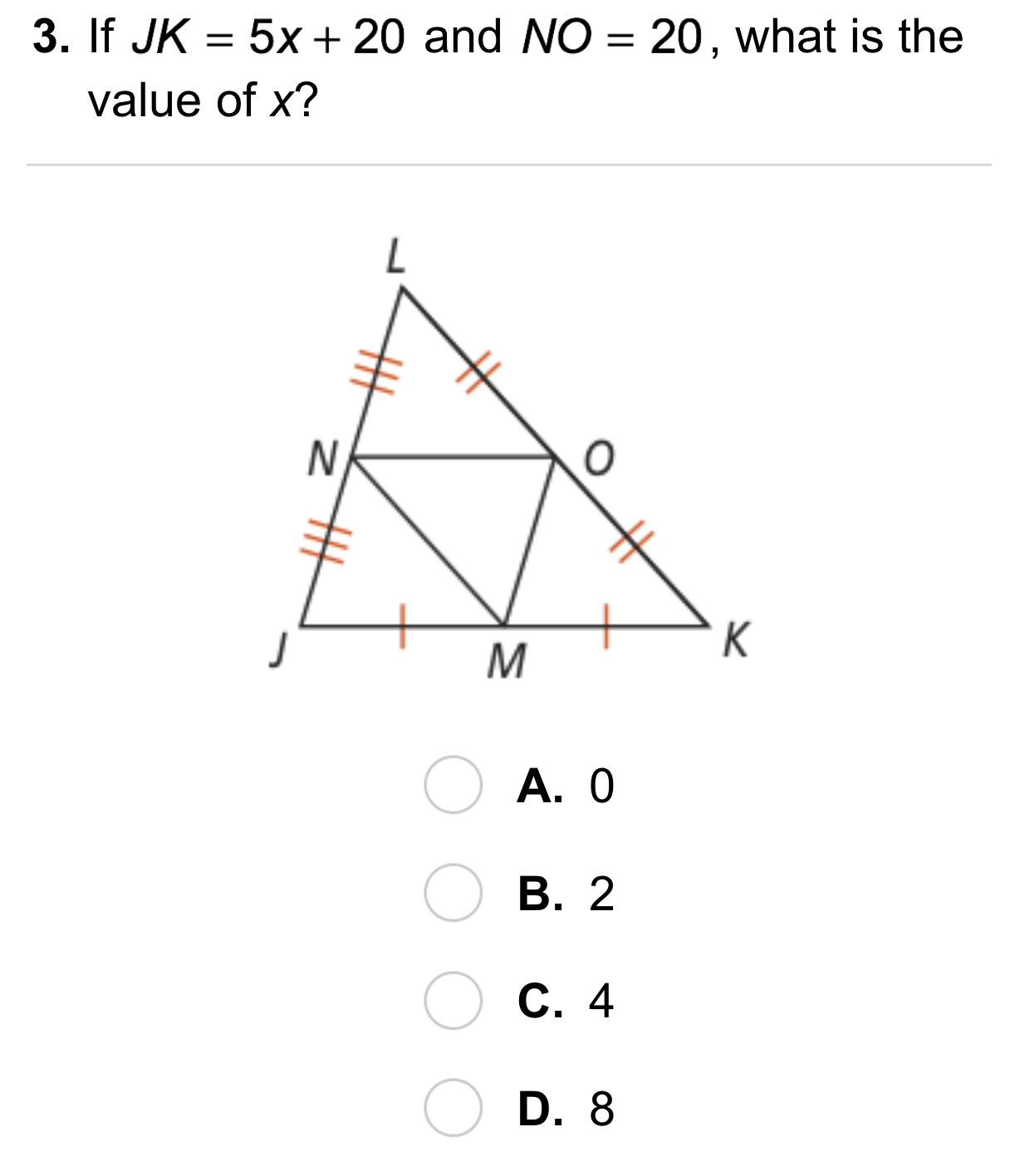
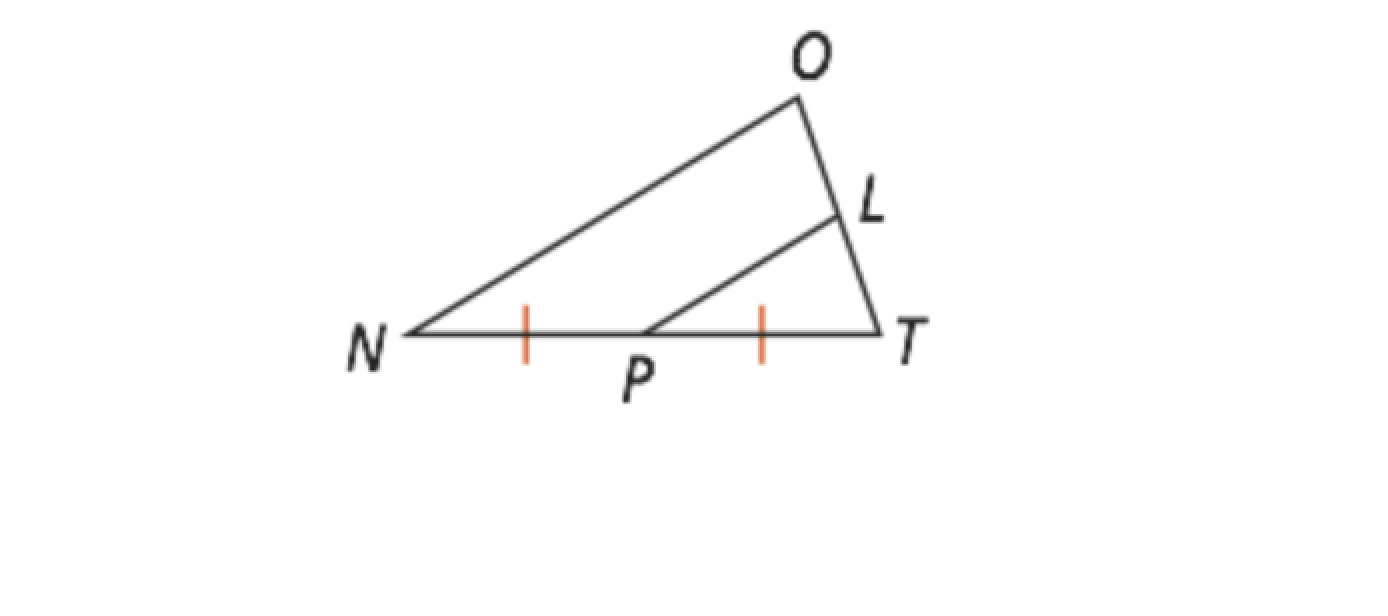
Geometry 5-1 Complete Lesson: Midsegments of Triangles

By Matt Richardson
Last updated about 3 years ago
22 Questions
Note from the author:
A complete formative lesson with embedded slideshow, mini lecture screencasts, checks for understanding, practice items, mixed review, and reflection. I create these assignments to supplement each lesson of Pearson's Common Core Edition Algebra 1, Algebra 2, and Geometry courses. See also mathquest.net and twitter.com/mathquestEDU.

Solve It! What is the relationship between MP and AB? How do you know?

Solve It! What conjecture can you make about the relationship between LN and AB?

Problem 1 Got It? In triangle XYZ, A is the midpoint of segment XY, B is the midpoint of segment YZ, and C is the midpoint of segment ZX.

Represent this information in a diagram on the canvas.

Problem 1 Got It? In triangle XYZ, A is the midpoint of segment XY, B is the midpoint of segment YZ, and C is the midpoint of segment ZX.

Match the three pairs of parallel segments.

  • segment AB
  • segment BC
  • segment CA
  • segment XY
  • segment YZ
  • segment ZX

Problem 1 Got It? Reasoning: What is m∠VUO in the figure? Enter only a number.

Problem 2 Got It?

Problem 3 Got It?

Vocabulary: How does the term midsegment describe the segments discussed in this lesson?

Reasoning: If two noncollinear segments in the coordinate plane have slope 3, what can you conclude?

Error Analysis: A student sees the figure and makes the following conclusion.
What is the error in the student's reasoning?

Review Lesson 4-7: ∠1 ≅ ∠2. Match congruent triangles from the figure.

  • △DFB
  • △DFA
  • △EBA
  • △CFA
  • △EFA
  • △DCA
  • △EFC
  • △BFA

Review Lesson 1-5: Segment TM bisects ∠STU so that m∠STU = 5x + 4 and m∠MTU = 6x - 2.

Sketch a diagram to represent the given information.

Review Lesson 1-5: Segment TM bisects ∠STU so that m∠STU = 5x + 4 and m∠MTU = 6x - 2.

Find the value of x.

Review Lesson 1-5: Segment TM bisects ∠STU so that m∠STU = 5x + 4 and m∠MTU = 6x - 2.

Find the m∠STU .

Review Lesson 1-6: Complete the following steps on the embedded GeoGebra Geometry utility above:
1. Construct acute ∠E
2. Construct the bisector of ∠E

Screenshot your construction and upload it to the canvas.
Remember to leave any evidence that indicates your method of construction.

Reflection: Math Success