Illustrative Math - Algebra 2 - Unit 2 - Lesson 2
By Formative Library
starstarstarstarstarstarstarstarstarstar
Last updated 27 days ago
10 Questions
1 point
1
Question 1
1.
Select all polynomial expressions that are equivalent to 6x^{4}+4x^{3}-7x^{2}+5x+8.
Select all polynomial expressions that are equivalent to 6x^{4}+4x^{3}-7x^{2}+5x+8.
…

1 point
1
Question 2
2.
1 point
1
Question 3
3.
1 point
1
Question 4
4.
Consider the polynomial function p given by p(x)=5x^{3}+8x^{2}-3x+1. Evaluate the function at x=-2.
Consider the polynomial function p given by p(x)=5x^{3}+8x^{2}-3x+1. Evaluate the function at x=-2.
…
1 point
1
Question 5
5.
An open-top box is formed by cutting squares out of a 5 inch by 7 inch piece of paper and then folding up the sides. The volume V(x) in cubic inches of this type of open-top box is a function of the side length x in inches of the square cutouts and can be given by V(x)=(7-2x)(5-2x)(x). Rewrite this equation by expanding the polynomial.
An open-top box is formed by cutting squares out of a 5 inch by 7 inch piece of paper and then folding up the sides. The volume V(x) in cubic inches of this type of open-top box is a function of the side length x in inches of the square cutouts and can be given by V(x)=(7-2x)(5-2x)(x). Rewrite this equation by expanding the polynomial.
…

1 point
1
Question 6
6.
1 point
1
Question 7
7.
1 point
1
Question 8
8.
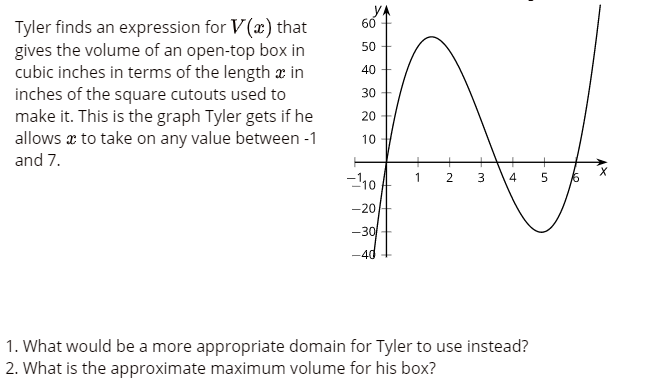
1 point
1
Question 9
9.
1 point
1
Question 10
10.
This lesson is from Illustrative Mathematics. Algebra 2, Unit 2, Lesson 2. Internet. Available from https://curriculum.illustrativemathematics.org/HS/teachers/3/2/2/index.html ; accessed 27/July/2021.
IM Algebra 1, Geometry, Algebra 2 is © 2019 Illustrative Mathematics. Licensed under the Creative Commons Attribution 4.0 International License (CC BY 4.0).
The Illustrative Mathematics name and logo are not subject to the Creative Commons license and may not be used without the prior and express written consent of Illustrative Mathematics.
These materials include public domain images or openly licensed images that are copyrighted by their respective owners. Openly licensed images remain under the terms of their respective licenses. See the image attribution section for more information.