Illustrative Math - Algebra 2 - Unit 4 - Lesson 13
By Formative Library
starstarstarstarstarstarstarstarstarstar
Last updated 27 days ago
14 Questions

1 point
1
Question 1
1.
1 point
1
Question 2
2.
1 point
1
Question 3
3.
1 point
1
Question 4
4.
The revenue of a technology company, in thousands of dollars, can be modeled with an exponential function whose starting value is $395,000 where time t is measured in years after 2010.
Which function predicts exactly 1.2% of annual growth: R(t)=395*e^{0.012t} or S(t)=395*(1.012)^{t}? Explain your reasoning.
The revenue of a technology company, in thousands of dollars, can be modeled with an exponential function whose starting value is $395,000 where time t is measured in years after 2010.
Which function predicts exactly 1.2% of annual growth: R(t)=395*e^{0.012t} or S(t)=395*(1.012)^{t}? Explain your reasoning.
…
1 point
1
Question 5
5.
How are the functions f and g given by f(x)=(1.05)^{x} and g(x)=e^{0.05x} similar?
How are they different?
How are the functions f and g given by f(x)=(1.05)^{x} and g(x)=e^{0.05x} similar?
How are they different?
…
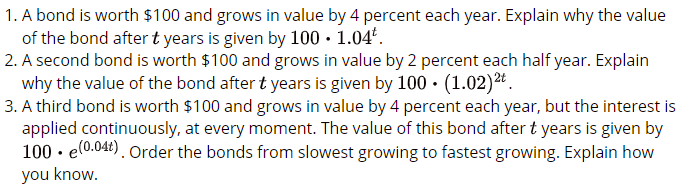
1 point
1
Question 6
6.
1 point
1
Question 7
7.
1 point
1
Question 8
8.
1 point
1
Question 9
9.
The population of a country is growing exponentially, doubling every 50 years.
What is the annual growth rate? Explain or show your reasoning.
The population of a country is growing exponentially, doubling every 50 years.
What is the annual growth rate? Explain or show your reasoning.
…
1 point
1
Question 10
10.
Which expression has a greater value: log_{3} \frac{1}{3} or log_{b} \frac{1}{b}?
Explain how you know.
Which expression has a greater value: log_{3} \frac{1}{3} or log_{b} \frac{1}{b}?
Explain how you know.
…

1 point
1
Question 11
11.
1 point
1
Question 12
12.
1 point
1
Question 13
13.
1 point
1
Question 14
14.
Select all true statements about the number e.
Select all true statements about the number e.
…
This lesson is from Illustrative Mathematics. Algebra 2, Unit 4, Lesson 13. Internet. Available from https://curriculum.illustrativemathematics.org/HS/teachers/3/4/13/index.html ; accessed 27/July/2021.
IM Algebra 1, Geometry, Algebra 2 is © 2019 Illustrative Mathematics. Licensed under the Creative Commons Attribution 4.0 International License (CC BY 4.0).
The Illustrative Mathematics name and logo are not subject to the Creative Commons license and may not be used without the prior and express written consent of Illustrative Mathematics.
These materials include public domain images or openly licensed images that are copyrighted by their respective owners. Openly licensed images remain under the terms of their respective licenses. See the image attribution section for more information.