Illustrative Math - Algebra 2 - Unit 3 - Lesson 15
By Formative Library
starstarstarstarstarstarstarstarstarstar
Last updated 27 days ago
16 Questions
1 point
1
Question 1
1.
Select all the expressions that are equivalent to (3-5i)(-8+2i).
Select all the expressions that are equivalent to (3-5i)(-8+2i).
1 point
1
Question 2
2.
Explain or show how to write (20-i)(8+4i) in the form a+bi, where a and b are real numbers.
Explain or show how to write (20-i)(8+4i) in the form a+bi, where a and b are real numbers.
1 point
1
Question 3
3.
Without going through all the trouble of writing the left side in the form a+bi, how could you tell that this equation is false?
(-9+2i)(10-13i)=-68-97i
Without going through all the trouble of writing the left side in the form a+bi, how could you tell that this equation is false?
(-9+2i)(10-13i)=-68-97i

1 point
1
Question 4
4.
1 point
1
Question 5
5.
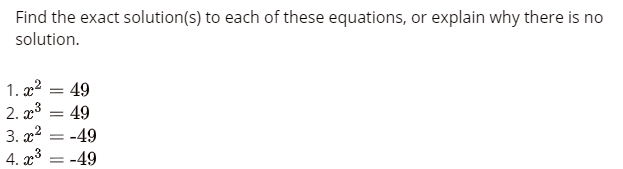
1 point
1
Question 6
6.
1 point
1
Question 7
7.
1 point
1
Question 8
8.
1 point
1
Question 9
9.
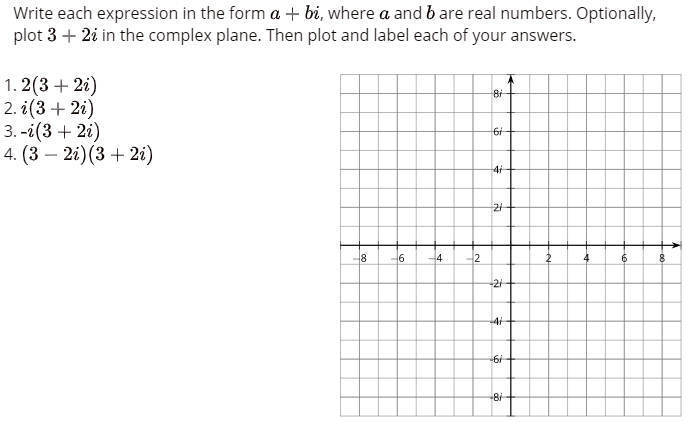
1 point
1
Question 10
10.
1 point
1
Question 11
11.
1 point
1
Question 12
12.
1 point
1
Question 13
13.
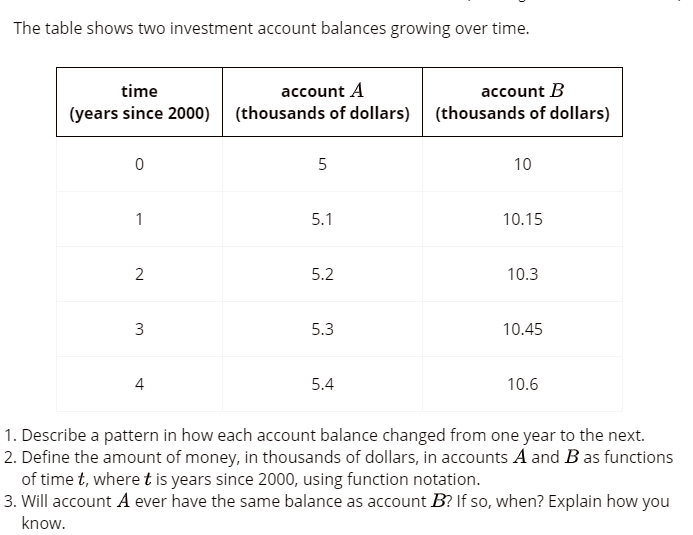
1 point
1
Question 14
14.
1 point
1
Question 15
15.
1 point
1
Question 16
16.
This lesson is from Illustrative Mathematics. Algebra 2, Unit 3, Lesson 15. Internet. Available from https://curriculum.illustrativemathematics.org/HS/teachers/3/3/15/index.html ; accessed 27/July/2021.
IM Algebra 1, Geometry, Algebra 2 is © 2019 Illustrative Mathematics. Licensed under the Creative Commons Attribution 4.0 International License (CC BY 4.0).
The Illustrative Mathematics name and logo are not subject to the Creative Commons license and may not be used without the prior and express written consent of Illustrative Mathematics.
These materials include public domain images or openly licensed images that are copyrighted by their respective owners. Openly licensed images remain under the terms of their respective licenses. See the image attribution section for more information.