Beer's Law Simulation for AP Chemistry
By Carson Dobrin
starstarstarstarstarstarstarstarstarstar
Last updated over 4 years ago
24 Questions
Note from the author:
Activity to accompany Beer's Law PhET. Adapted from activity by Brian Brown.
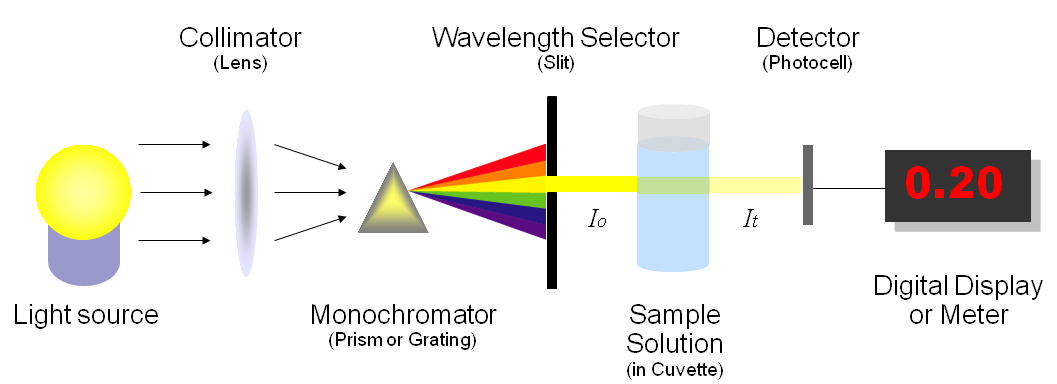
What is a spectrophotometer?
Spectrophotometers are used by chemists to study how much light at a specific wavelength is absorbed as it passes through a colored solution. (illustration by Heesung Shim)
Spectrophotometers (and colorimeters) use light absorbance to determine the concentration of colored solutions. At lower concentrations, the following equation, known as the Beer-Lambert law (Beer’s Law), is valid:
The Beer’s Law simulator below was developed by PhET Interactive Simulations by the University of Colorado Boulder. This is an online spectrophotometry simulator that we can use to study Beer’s Law and how we can use this law to determine the concentration of a solution using experimental data.
You can also open the simulation in another tab using this link:
https://phet.colorado.edu/sims/html/beers-law-lab/latest/beers-law-lab_en.htm
Part 1: Choosing the right Wavelength
- Reset the simulation.
- Chose nickel (II) chloride as the solute, turn on the light, and set the meter to absorbance (type of measurement used in Beer’s Law calculations).
1
1.
What is the preset wavelength? ___________
What is the preset wavelength? ___________
1
2.
To see why this wavelength was chosen, change the wavelength setting to variable. Move the slider left and right of the preset wavelength and see what happens to the absorbance. The preset wavelength corresponds to the _______________________ absorbance. The is done to minimize percent error.
To see why this wavelength was chosen, change the wavelength setting to variable. Move the slider left and right of the preset wavelength and see what happens to the absorbance. The preset wavelength corresponds to the _______________________ absorbance. The is done to minimize percent error.
1
3.
The best wavelength to use in a Beer’s law lab is usually a color that is complementary to the color of the solution. What do you think the light color will be for a solution with a yellow color like potassium chromate? _______________________ (If you don’t know the color wheel from grade school art, google what color is complementary to yellow.)
The best wavelength to use in a Beer’s law lab is usually a color that is complementary to the color of the solution. What do you think the light color will be for a solution with a yellow color like potassium chromate? _______________________ (If you don’t know the color wheel from grade school art, google what color is complementary to yellow.)
1
4.
Change the solute to potassium dichromate. Was your prediction correct? __
Change the solute to potassium dichromate. Was your prediction correct? __
Section 2: Path Length
- Reset the simulation. Turn on the light and set the meter to absorbance.
- Move the ruler to measure the cuvette width.
1
5.
Measure (to proper precision) the cuvette width? ________ cm This is the measured path length (b) in the Beer’s Law equation. Do not include units in your answer and remember the measurement rule - you can estimate to 1 digit better than the smallest division!
Measure (to proper precision) the cuvette width? ________ cm This is the measured path length (b) in the Beer’s Law equation. Do not include units in your answer and remember the measurement rule - you can estimate to 1 digit better than the smallest division!
1
6.
Grab and drag the right side of the cuvette and make it larger. What happens to the absorbance? ___________________ (increases, decreases or stays constant)
Grab and drag the right side of the cuvette and make it larger. What happens to the absorbance? ___________________ (increases, decreases or stays constant)
1
7.
Why? Because the light has to travel thru more solution, it will collide with ________________ solute particles causing a _______________ absorbance.
Why? Because the light has to travel thru more solution, it will collide with ________________ solute particles causing a _______________ absorbance.
Section 3: Other Variables
- Reset the simulation.
- Turn on the light and set the meter to measure absorbance.
- Set the wavelegth to "varied" and set it to 500 nm.
1
8.
At the same concentration (100 mM which is 0.1 M), should the measured absorbance at 500 nm for three red solutions (drink mix, cobalt (II) nitrate and cobalt (II) chloride) be similar.
At the same concentration (100 mM which is 0.1 M), should the measured absorbance at 500 nm for three red solutions (drink mix, cobalt (II) nitrate and cobalt (II) chloride) be similar.
1
9.
What is the measured absorbance at 500 nm for drink mix?
What is the measured absorbance at 500 nm for drink mix?
1
10.
What is the measured absorbance at 500 nm for cobalt (II) nitrate?
What is the measured absorbance at 500 nm for cobalt (II) nitrate?
1
11.
What is the measured absorbance at 500 nm for cobalt (II) chloride)?
What is the measured absorbance at 500 nm for cobalt (II) chloride)?
1
12.
These absorbances vary because while each of the solutions have the same concentration, path length, and wavelength of monochromatic light, they will all have different _________________________________ (which is a constant for that depends upon the identity of the solution in Beer's Law calculations).
These absorbances vary because while each of the solutions have the same concentration, path length, and wavelength of monochromatic light, they will all have different _________________________________ (which is a constant for that depends upon the identity of the solution in Beer's Law calculations).
Section 4: Concentration
Reset the simulation. Turn on the light and set the meter to absorbance.
1
13.
For 0.10 M drink mix at the preset wavelength, what is the absorbance? __________
For 0.10 M drink mix at the preset wavelength, what is the absorbance? __________
1
14.
If the concentration is increased, what should happen to the absorbance? ____________________
If the concentration is increased, what should happen to the absorbance? ____________________
1
15.
Why? Because the light has to travel thru a more concentrated solution, this will cause the photons of light to collide with more particles causing _______________ photons to be absorbed which causes a _______________ absorbance
Why? Because the light has to travel thru a more concentrated solution, this will cause the photons of light to collide with more particles causing _______________ photons to be absorbed which causes a _______________ absorbance
1
16.
What is the absorbance of drink mix if the concentration is 0.15 M? __________
What is the absorbance of drink mix if the concentration is 0.15 M? __________
1
17.
What is the absorbance of drink mix if the concentration is 0.40 M? __________
What is the absorbance of drink mix if the concentration is 0.40 M? __________
Section 5: Beer's Law Graph
The following data was obtained for cobalt (II) nitrate.
concentration: 0.10 absorbance: 0.47
concentration: 0.15 absorbance: 0.71
concentration: 0.40 absorbance: 1.89
1
18.
When the same solution and cuvette are used, the absorptivity coefficient and path length will be _________________ (constant or variable).
When the same solution and cuvette are used, the absorptivity coefficient and path length will be _________________ (constant or variable).
1
19.
This leaves absorption and concentration as the only remaining variables in Beer’s Law. A graph of these two variables will be __________________.
This leaves absorption and concentration as the only remaining variables in Beer’s Law. A graph of these two variables will be __________________.
1
20.
Open google sheets and plot the data. Using the data above graph the absorbance versus concentration, plot the points. (y- absorbance, x- concentration). Is your graph linear?concentration: 0.10 absorbance: 0.47concentration: 0.15 absorbance: 0.71concentration: 0.40 absorbance: 1.89
Open google sheets and plot the data. Using the data above graph the absorbance versus concentration, plot the points. (y- absorbance, x- concentration). Is your graph linear?
concentration: 0.10 absorbance: 0.47
concentration: 0.15 absorbance: 0.71
concentration: 0.40 absorbance: 1.89
1
21.
What is the slope of the best fit line (record answer to 2 decimal places)? __________ (units will be 1/M but do not include them in your reported answer)
What is the slope of the best fit line (record answer to 2 decimal places)? __________ (units will be 1/M but do not include them in your reported answer)
Now we know that slope...
1
22.
Using the rounded value from #21 calculate the absorptivity coefficient (Ɛ) of cobalt (II) nitrate. The unit will be 1/cmM but do not include units in your answer). (Hint: the math should be very easy)
Using the rounded value from #21 calculate the absorptivity coefficient (Ɛ) of cobalt (II) nitrate. The unit will be 1/cmM but do not include units in your answer). (Hint: the math should be very easy)
Part 6: More Graphing
This is one way that you can use Beer's Law to determine the concentration of a solution. With a few standard solutions, you can establish a simple method to predict other concentrations based upon their absorbance. You can interpolate/extrapolate from the graph or use the equation for the line to predict the concentration.
1
23.
What would the concentration be of an unknown sample that had an absorbance value of 1?
What would the concentration be of an unknown sample that had an absorbance value of 1?
1
24.
What would the concentration be of an unknown sample that had an absorbance value of .4?
What would the concentration be of an unknown sample that had an absorbance value of .4?
Adapted from an activity created by Brian Brown at Hononegah High School.