3.0 - Acceleration Homework
By Caleb Guesno
starstarstarstarstar
Last updated about 2 years ago
31 Questions
Note from the author:
Information to create this formative was gathered from:
https://www.khanacademy.org/science/physics/one-dimensional-motion/acceleration-tutorial/a/acceleration-article
Information to create this formative was gathered from:
https://www.khanacademy.org/science/physics/one-dimensional-motion/acceleration-tutorial/a/acceleration-article
What does acceleration mean?
Compared to displacement and velocity, acceleration is like the angry, fire-breathing dragon of motion variables. It can be violent; some people are scared of it; and if it's big, it forces you to take notice. That feeling you get when you're sitting in a plane during take-off, or slamming on the brakes in a car, or turning a corner at a high speed in a go kart are all situations where you are accelerating.
Acceleration is the name we give to any process where the velocity changes. Since velocity is a speed and a direction, there are only two ways for you to accelerate: change your speed or change your direction—or change both.
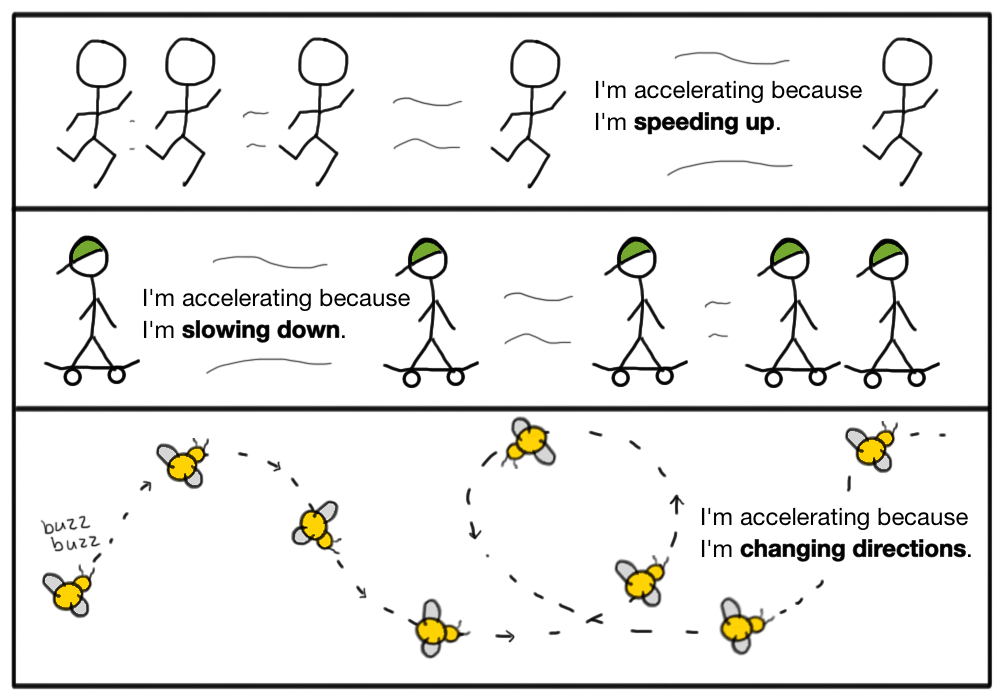
If you’re not changing your speed and you’re not changing your direction, then you simply cannot be accelerating—no matter how fast you’re going. So, a jet moving with a constant velocity at 800 miles per hour along a straight line has zero acceleration, even though the jet is moving really fast, since the velocity isn’t changing. When the jet lands and quickly comes to a stop, it will have acceleration since it’s slowing down.
Or, you can think about it this way. In a car you could accelerate by hitting the gas or the brakes, either of which would cause a change in speed. But you could also use the steering wheel to turn, which would change your direction of motion. Any of these would be considered an acceleration since they change velocity.
What's the formula for acceleration?
To be specific, acceleration is defined to be the rate of change of the velocity:
acceleration = change in velocity / change in time
a= Δv / Δt
or
a= (vfinal - vinitial) / (tfinal - tinitial)
The above equation says that the acceleration, a, is equal to the difference between the initial and final velocities, vf - vi, divided by the time, tΔ, it took for the velocity to change from start vi to finish vf
Note that the units for acceleration are m/s/s which can also be written as m/s2
I have to warn you that acceleration is one of the first really tricky ideas in physics. The problem isn’t that people lack an intuition about acceleration.
The incorrect intuition usually goes a little something like this: “Acceleration and velocity are basically the same thing, right?” Wrong. People often erroneously think that if the velocity of an object is large, then the acceleration must also be large. Or they think that if the velocity of an object is small, it means that acceleration must be small. But that “just ain’t so”. The value of the velocity at a given moment does not determine the acceleration. In other words, I can be changing my velocity at a high rate regardless of whether I'm currently moving slow or fast.
People think, “If the acceleration is negative, then the object is slowing down, and if the acceleration is positive, then the object is speeding up, right?”
Wrong.
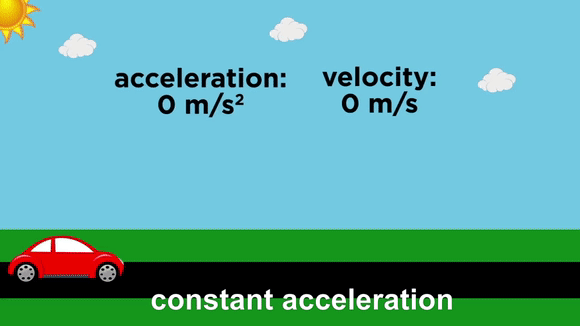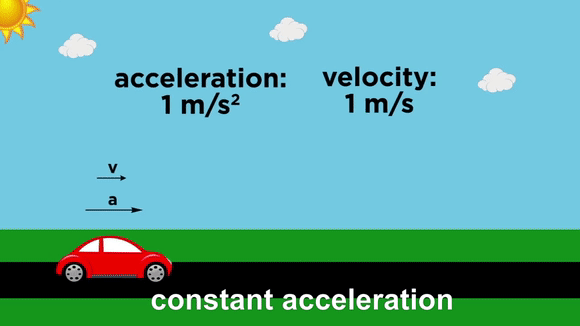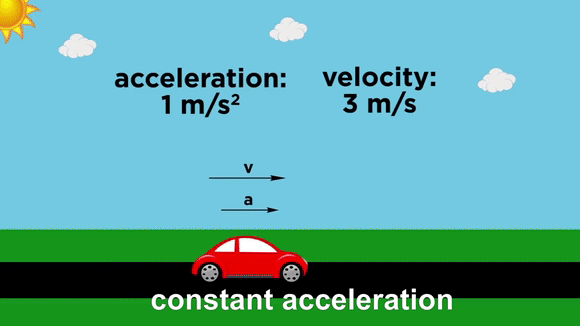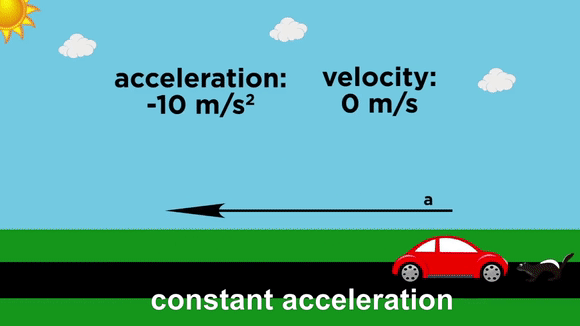
An object with negative acceleration could be speeding up, and an object with positive acceleration could be slowing down. How is this so? Consider the fact that acceleration is a vector that points in the same direction as the change in velocity.
That means that the direction of the acceleration determines whether you will be adding to or subtracting from the velocity. Mathematically, a negative acceleration means you will subtract from the current value of the velocity, and a positive acceleration means you will add to the current value of the velocity. Subtracting from the value of the velocity could increase the speed of an object if the velocity was already negative to begin with since it would cause the magnitude to increase.
If acceleration points in the same direction as the velocity, the object will be speeding up. And if the acceleration points in the opposite direction of the velocity, the object will be slowing down. Check out the accelerations in the diagram below, where a car accidentally drives into the mud—which slows it down—or chases down a donut—which speeds it up. Assuming rightward is positive, the velocity is positive whenever the car is moving to the right, and the velocity is negative whenever the car is moving to the left. The acceleration points in the same direction as the velocity if the car is speeding up, and in the opposite direction if the car is slowing down.
Another way to say this is that if the acceleration has the same sign as the velocity, the object will be speeding up. And if the acceleration has the opposite sign as the velocity, the object will be slowing down.