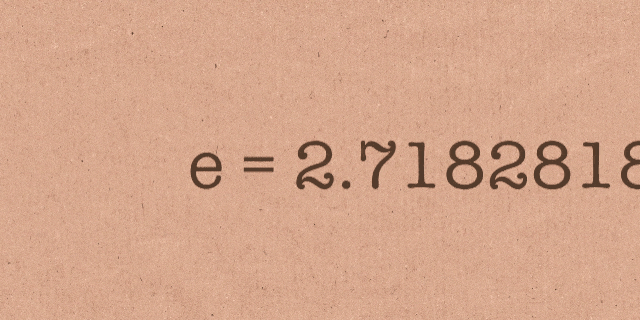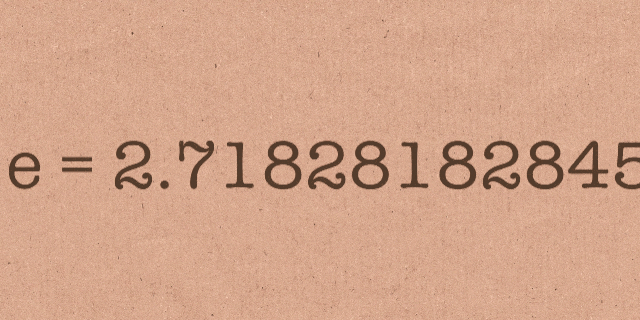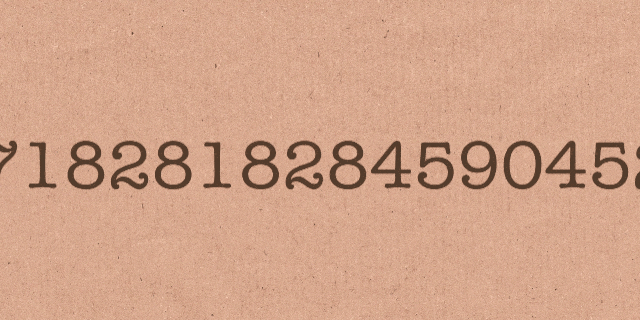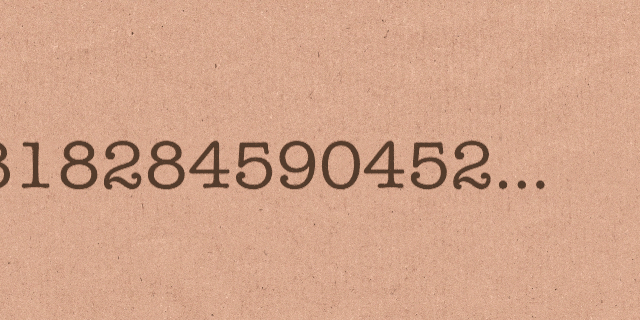Exponential Slope Investigation
By David Kwan
starstarstarstarstarstarstarstarstarstar
Last updated over 6 years ago
21 Questions
Note from the author:
This Formative is by: Brian Gervase
This is a guided discovery investigation that leads students to the number 'e' via slope patterns in functions. Embedded Geogebra labs create a place for students to play!
In this investigation, you will:
1) Identify patterns in polynomial functions involving slope.
2) Make note of significant slope difference between polynomial and exponential growth.
3) Identify a specific base value for exponential functions that has satisfying qualities!
1 point
1
Question 1
1.
The slope of the linear function above depends on the value of x at which you measure the slope.
The slope of the linear function above depends on the value of x at which you measure the slope.
1 point
1
Question 2
2.
What is the slope of the curve above when x = 3.7 ?
What is the slope of the curve above when x = 3.7 ?
1 point
1
Question 3
3.
The slope of the quadratic function above depends on the value of x at which you measure the slope.
The slope of the quadratic function above depends on the value of x at which you measure the slope.
1 point
1
Question 4
4.
Can you identify a pattern between the slope of the curve and x-value at which the slope is measured? Describe the pattern you observe.
Can you identify a pattern between the slope of the curve and x-value at which the slope is measured? Describe the pattern you observe.
1 point
1
Question 5
5.
What will the slope of the above quadratic equation be when x = 50?
What will the slope of the above quadratic equation be when x = 50?
1 point
1
Question 6
6.
At what x-value does the curve have a slope of 5?
At what x-value does the curve have a slope of 5?
1 point
1
Question 7
7.
The slope of the cubic function above depends on the value of x at which you measure the slope.
The slope of the cubic function above depends on the value of x at which you measure the slope.
1 point
1
Question 8
8.
Can you identify a pattern between the slope of the curve and x-value at which the slope is measured? Describe the pattern you observe.
Can you identify a pattern between the slope of the curve and x-value at which the slope is measured? Describe the pattern you observe.
1 point
1
Question 9
9.
What will the slope of the above quadratic equation be when x = 20?
What will the slope of the above quadratic equation be when x = 20?
1 point
1
Question 10
10.
At what x-value does the graph have a slope of 5? You may want to use a calculator to help make your prediction! Give your answer to THREE decimal places.
At what x-value does the graph have a slope of 5? You may want to use a calculator to help make your prediction! Give your answer to THREE decimal places.
1 point
1
Question 11
11.
Make an observation about the slope of the curve above. Based on the slope pattern, can you predict what the equation of the function is? Explain how you might know.
Make an observation about the slope of the curve above. Based on the slope pattern, can you predict what the equation of the function is? Explain how you might know.
1 point
1
Question 12
12.
At what x-value is the slope of the curve equal to 10? You may need to use a calculator! Round your answer to three decimal places.
At what x-value is the slope of the curve equal to 10? You may need to use a calculator! Round your answer to three decimal places.
1 point
1
Question 13
13.
Which statement best describes the slope of the exponential function?
Which statement best describes the slope of the exponential function?
1 point
1
Question 14
14.
Clicking the "Interesting Fact" button shows the ratio of the slope and the current y-value. Explain what this fact seems to imply.
Clicking the "Interesting Fact" button shows the ratio of the slope and the current y-value. Explain what this fact seems to imply.
1 point
1
Question 15
15.
Use the interesting fact and a calculator to estimate the slope of this curve when the y-value is 20. Give your answer to one decimal place.
Use the interesting fact and a calculator to estimate the slope of this curve when the y-value is 20. Give your answer to one decimal place.
1 point
1
Question 16
16.
Move the purple slider to x = 0. What is the slope of the curve at this point?
Move the purple slider to x = 0. What is the slope of the curve at this point?
1 point
1
Question 17
17.
Move the black slider above and show the "interesting fact". What value on the black slider creates the exact same exponential function from the previous set of questions.
Move the black slider above and show the "interesting fact". What value on the black slider creates the exact same exponential function from the previous set of questions.
1 point
1
Question 18
18.
Change the base of the exponential function to 5 and then slide the purple slider. What is the constant ratio of the slope and the y-value for this curve?
Change the base of the exponential function to 5 and then slide the purple slider. What is the constant ratio of the slope and the y-value for this curve?
1 point
1
Question 19
19.
Play with various values for the base. Based on what you observe, which of the statement(s) are accurate?
Play with various values for the base. Based on what you observe, which of the statement(s) are accurate?
So far we have discovered that the slope of an exponential function is VERY closely related to the y-value of the function at that instant. Since the y-value of a function is really the "value" of the function, we can say that exponential functions have slope that depends on the current value of the function.
1 point
1
Question 20
20.
There is a common saying, "It takes money to make money!"
Joe has $100 he is investing and earning 10% per year. Karl has $10,000 and is also earning 10% per year.
Consider the first couple years of Joe and Karl earning interest on their money. Explain how Joe and Karl are representative of the above saying.
There is a common saying, "It takes money to make money!"
Joe has $100 he is investing and earning 10% per year. Karl has $10,000 and is also earning 10% per year.
Consider the first couple years of Joe and Karl earning interest on their money. Explain how Joe and Karl are representative of the above saying.
So far we have observed that exponential fucntions have very similar form and shape and vary only in steepness. We have also observed that the steepness seems to be a constant factor that increases at the base increases.
How cool would it be if we found the PERFECT function where the slope and the y-value were exactly equal! That is, the special ratio for this exponential would be 1 !
Go back to the above graph and move the black slider. Take note that the ratio is less than 1 for base = 2 but greater than one for base = 3. For all bases bigger than 3 the ratio just keeps getting bigger.
Let's take a closer look BETWEEN the base being 2 and 3 below.
1 point
1
Question 21
21.
Play with the black slider until you make the ratio equal to 1. What value of the base achieves this?
Play with the black slider until you make the ratio equal to 1. What value of the base achieves this?
Congratulations! You have just had an encounter with one of mathematics most interesting irrational numbers - simply called "e".
The exponential function with e as the base turns out to yield some beautiful mathematical symmetries and makes calculus smoother and cleaner. Since ALL exponentials have the same general shape, we typically use the base of 'e' to be "THE exponential function" used by default.