Investigating Volume of Cylinders
By David Kwan
starstarstarstarstarstarstarstarstarstar
Last updated almost 7 years ago
27 Questions
Note from the author:
This Formative is by: Rebecca Mann
This is an activity introducing volume in a high school geometry class. Using a 3-Act Math Task and an Illuminations Task, students conceptualize the idea of volume and develop the volume of cylinders.
Watch the Act 1: Popcorn Picker Video Below.
1 point
1
Question 1
1.
Based on the video, what question(s) could we explore?
Based on the video, what question(s) could we explore?

1 point
1
Question 2
2.
Which container will hold more popcorn?
Which container will hold more popcorn?
1 point
1
Question 3
3.
What information do you need to explore the question?
What information do you need to explore the question?
Act 2: POPCORN CYLINDER TASK
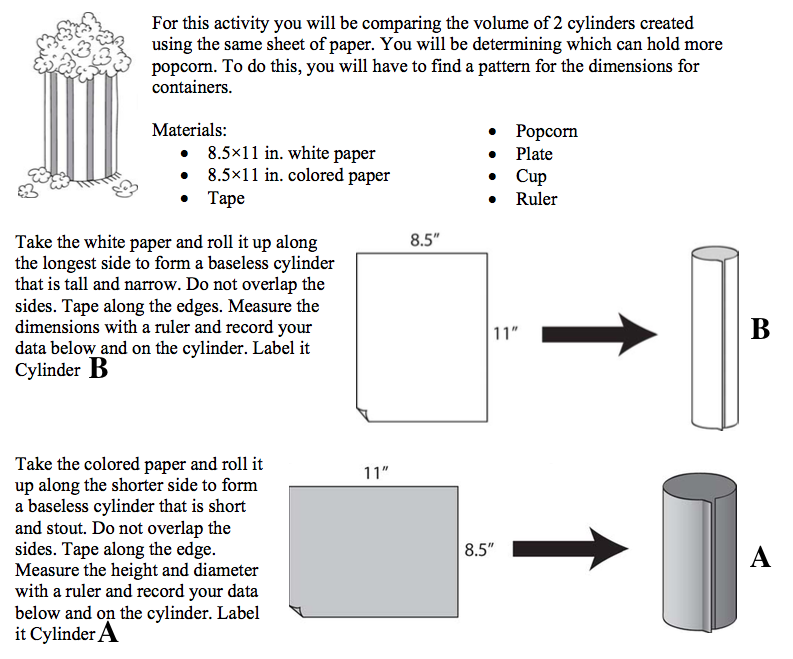
1 point
1
Question 4
4.
1 point
1
Question 5
5.
1 point
1
Question 6
6.
1 point
1
Question 7
7.
1 point
1
Question 8
8.
1 point
1
Question 9
9.
1 point
1
Question 10
10.
Do you think the two cylinders will hold the same amount? Do you think one will hold more than the other one? Which one? Why?
Do you think the two cylinders will hold the same amount? Do you think one will hold more than the other one? Which one? Why?
Come get your popcorn when everyone in your group has completed everything up to this point.
***Read Carefully!!! Place Cylinder A on the paper plate with Cylinder B inside it. Use your cup to pour popcorn into Cylinder B until it is full. Carefully, lift Cylinder B so that the popcorn falls into Cylinder A.
1 point
1
Question 11
11.
Describe what happened. Is Cylinder A full, not full, or overflowing?
Describe what happened. Is Cylinder A full, not full, or overflowing?
As you share your popcorn snack, answer the following questions.
1 point
1
Question 12
12.
Was your prediction correct? How do you know?
Was your prediction correct? How do you know?
1 point
1
Question 13
13.
If your prediction was incorrect, describe what actually happened.
If your prediction was incorrect, describe what actually happened.
Act 3: Popcorn Picker Solution
1 point
1
Question 14
14.
Can a rectangular piece of paper give you the same amount of popcorn no matter how you make the cylinder?
Can a rectangular piece of paper give you the same amount of popcorn no matter how you make the cylinder?
Watch the video below to see if your prediction is correct.
Deriving the Volume of a Cylinder
Move the slider to see how the cylinder is being filled on the GeoGebra applet below.
1 point
1
Question 15
15.
The applet above illustrates how the cylinder is being filled to specific heights. We can think of volume of a cylinder as stacking ____________________ to a specific height.
The applet above illustrates how the cylinder is being filled to specific heights. We can think of volume of a cylinder as stacking ____________________ to a specific height.
1 point
1
Question 16
16.
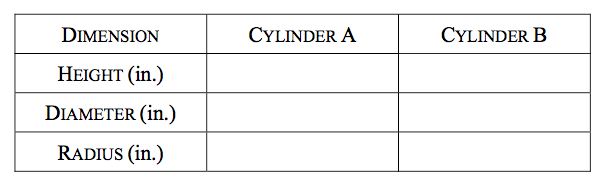
Calculate the volume of Cylinder A. Label your dimensions on the diagram.
Calculate the volume of Cylinder A. Label your dimensions on the diagram.
1 point
1
Question 17
17.
Calculate the volume of Cylinder B. Label your dimensions on the diagram.
Calculate the volume of Cylinder B. Label your dimensions on the diagram.
1 point
1
Question 18
18.
Explain why the cylinders do or do not hold the same amount of popcorn. Use the formula for the volume of a cylinder to guide your explanation.
Explain why the cylinders do or do not hold the same amount of popcorn. Use the formula for the volume of a cylinder to guide your explanation.
1 point
1
Question 19
19.
Which measurement impacts the volume of more: the radius or the height?
Which measurement impacts the volume of more: the radius or the height?
1 point
1
Question 20
20.
Why do you believe your response to #19 is true?
Why do you believe your response to #19 is true?
1 point
1
Question 21
21.
By how much would you have to decrease the height of the Cylinder A to make the volumes of the two cylinders equal?
By how much would you have to decrease the height of the Cylinder A to make the volumes of the two cylinders equal?
Cavalieri's Principle - GeoGebra Applet
Click the play button to begin the applet.
1 point
1
Question 22
22.
Why do the two solids contain the same amount of volume?
Why do the two solids contain the same amount of volume?
Check the box beside "Solids" on the applet.
1 point
1
Question 23
23.
What do you notice?
What do you notice?
1 point
1
Question 24
24.
This applet visually describes the Cavalieri's Principle. Based on what you have observed, what is Cavalieri's Principle in your own words?
This applet visually describes the Cavalieri's Principle. Based on what you have observed, what is Cavalieri's Principle in your own words?
CLOSER - BY YOURSELF. :)
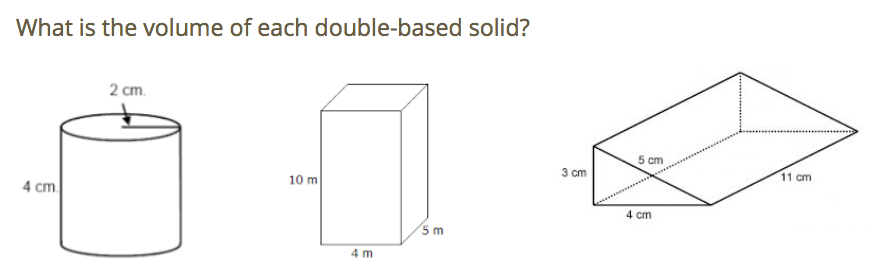
1 point
1
Question 25
25.
1 point
1
Question 26
26.
1 point
1
Question 27
27.